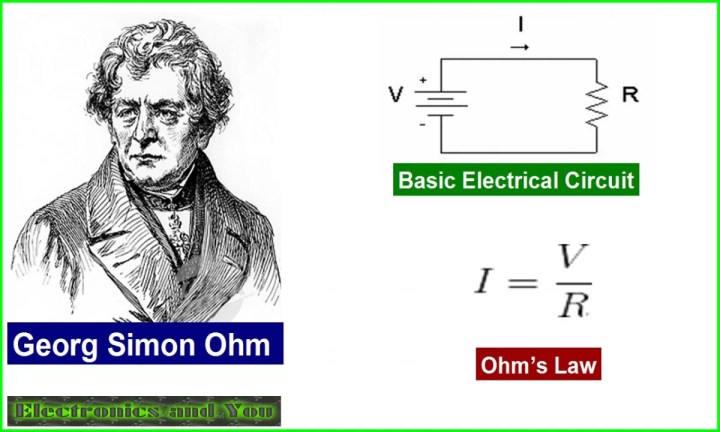
Ohm’s law is a fundamental principle in electrical engineering and electronics that describes the relationship between voltage, current and resistance in electrical and electronic circuits. First proposed by German physicist Georg Ohm in 1827, it underpins the operation of all manner of circuits, from simple DC circuits to modern microchips. This comprehensive guide will provide an in-depth look at Ohm’s law, its history, formulas, applications and limitations.

Origins and History of Ohm’s Law

In the early 19th century, the nature of the relationship between voltage, current and resistance was not well understood. Scientists knew that increasing voltage tended to increase current, but the exact relationship was unclear. After performing extensive experiments using high-precision equipment, Georg Ohm published his now famous law in a 1827 treatise called “The Galvanic Circuit Investigated Mathematically”.

Ohm summarized his findings in what is now known as Ohm’s law:

“The current strength produced by a given electromotive force is directly proportional to the conductor’s conductivity: the two poles of a galvanic battery are connected to the two ends of a wire whose length is 1 meter. I then find that the quantity of electricity that flows through this wire in one second is directly proportional to the electromotive force applied to the battery and inversely proportional to the resistance of the wire.”

This seminal work provided for the first time a precise mathematical equation that could predict and calculate electrical behavior, helping launch the modern field of circuit analysis. Prior to Ohm’s discovery, electronics amounted to little more than trial-and-error tinkering. Thomas Edison famously said “I am delighted to honor Georg Ohm, who by a single work has contributed more to electrical science than any man up to his time.”

The Relationship Between Voltage, Current and Resistance

The core idea of Ohm’s law is the relationship between voltage, current and resistance. Voltage (V) is the electromotive force or electrical pressure that causes electrons to flow and is measured in volts. Current (I) is the rate of flow of electrons in a circuit and is measured in amperes. Resistance (R) is a material’s opposition to electron flow and is measured in ohms.

Using the analogy of water flow in pipes, voltage is equivalent to water pressure, current is the water flow rate and resistance is the pipe size. Ohm’s core finding was that voltage and current are directly proportional, while current is inversely proportional to resistance. Doubling the voltage doubles the current, while doubling the resistance halves the current.

This relationship is encapsulated in the canonical equation for Ohm’s law:

V = I x R

Where:

V = Voltage in volts

I = Current in amps

R = Resistance in ohms

This simple but powerful formula allows calculation of any one variable when the other two are known. For example, if the voltage is 12V and the resistance is 4 ohms, the current must be 12/4 = 3 amperes. Ohm’s law opened up circuit analysis as an exact science.

Applications and Limitations of Ohm’s Law

Thanks to the simplicity of Ohm’s law, it can be readily applied to analyze and design all types of basic electrical DC circuits, including series, parallel and combination circuits. As Thomas Edison noted, it is perhaps the single most useful calculation in electronics. A few examples of common applications include:

• Calculating required resistor values for LED current limiting
• Determining expected current draw for fuses and power supplies
• Calculating effective resistance in parallel resistor networks
• Understanding short and open circuit faults
• Designing voltage divider circuits

However, it’s important to understand the limitations of Ohm’s law. It assumes a stable linear relationship that only applies to certain conditions:

• Only valid for DC circuits, not AC
• Applies to conductors with constant temperature
• Resistors must obey Ohm’s law (e.g. not semiconductor diodes)
• Current flow must be steady, not transient

As the famous scientist James Clerk Maxwell put it:

“Ohm’s law is of the same type as the statement that the force on a body is equal to its mass multiplied by its acceleration; a simple general law which is true only under certain restricted conditions.”

So in more complex circuits with changing signals and nonlinear electronic components, more advanced analysis is required. But for most basic DC circuits, Ohm’s law remains as relevant today as when Georg Ohm first discovered it nearly 200 years ago.

Using Ohm’s Law in Circuit Calculations

Let’s look at a few examples of how to apply Ohm’s law to solve practical circuit problems:

Example 1

If a circuit with a 6V battery passes 150mA of current, what is the resistance?

Using V = IR, we can calculate:

R = V/I R = 6V / 0.15A R = 40Ω

Example 2

A current of 500mA passes through a 47Ω resistor. What voltage develops across it?

Using V = IR, we can calculate:

V = I x R V = 0.5A x 47Ω V = 23.5V

Example 3

What resistance is needed to draw 300mA of current from a 9V supply?

Using R = V/I, we can calculate:

R = V/I R = 9V / 0.3A R = 30Ω

These examples demonstrate how Ohm’s law can be applied to practical circuit calculations using the three key variables of voltage, current and resistance. With just a little algebra, the voltage, current or resistance in a circuit can easily be determined.

Conclusion

In summary, Ohm’s law forms the basis for analysis of electrical circuits by relating voltage, current and resistance. First stated by Georg Ohm in 1827, this simple but powerful formula allows calculation of any one variable when the other two are known. It enables circuit designers to compute required resistor values, expected currents, voltage drops and more. While limited to linear circuits, it remains indispensable for designing and troubleshooting all types of basic DC electric circuits. Ohm’s elegant formula will undoubtedly continue providing the fundamental understanding of circuit behavior far into the future.